Massive Particles

Relatively larger particles are used in some systems like fluidized beds, where the inertial effect is not negligible anymore. For such massive particles, a dynamics presented in Ref. [16,17] is implemented:

$$\widetilde{\text{St}}\ \frac{{\rm d}\bm{U}}{{\rm d}t} = -\bm{U}+\bm{V} ,$$ (2.16)

$$\frac{{\rm d}\bm{x}}{{\rm d}t} = \bm{U} ,$$ (2.17)

where $\widetilde{\text{St}}$ is the effective Stokes number representing the inertial effect of the particle, $\bm{U}$ is the particle velocity, and $\bm{V}$ is the terminal velocity obtained by the mobility (or mixed) problem in this case given by

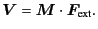$$\bm{V} = \bm{M}\cdot\bm{F}_{\text{ext}} .$$ (2.18)

The parameter ``stokes'' in the initialization script corresponds to $\widetilde{\text{St}}$.

In this dynamics, collisions among particles are implemented not by the contact force but by the momentum exchange for the contact pairs. The frequency of the contact-check is controlled by the parameter ``ncol''.